Blunders, viewed as aids to memory
-
-
slice.mit.edu
- 6
Filed Under
Recommended
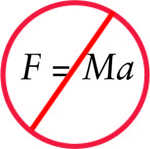 Professor Patrick Henry Winston ’65, SM ’67, PhD ’70
Professor Patrick Henry Winston ’65, SM ’67, PhD ’70I was rummaging around in the attic when I stumbled across my notes and quizzes from my first term, fifty years ago. I opened up the binder and there it was: the dreaded 8.01 quiz #2.
When I was a freshman, I always wrote down F = Ma, force is equal to mass times acceleration, as the first step toward solving 8.01 problems. Writing it down got the formula into my visual field, which generaly is a good idea, because visual problem solving is an important contributor to problem solving.
Alas, on that 8.01 quiz #2, writing F = Ma got me into big trouble.
This was the problem: an open railroad car rolls along a frictionless track at constant speed, v. Then, it starts to rain into the car. What force is required to keep the car going at constant speed?
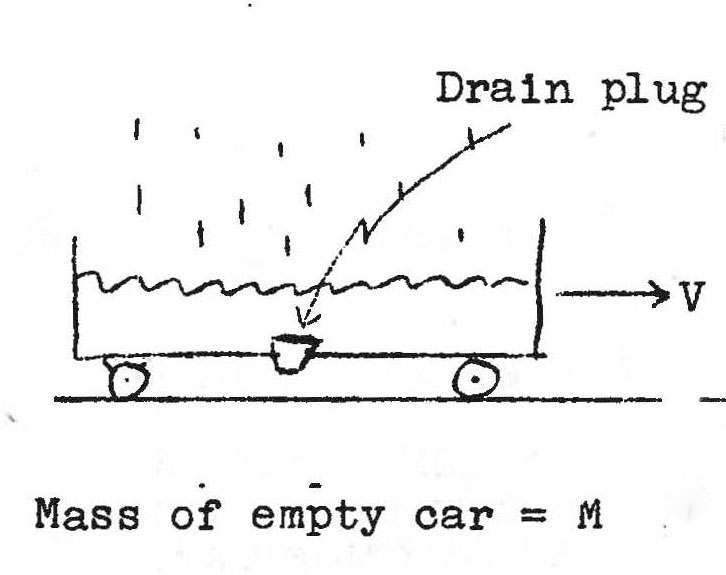
I concluded that each drop went from zero horizontal velocity to v instantaneously, but then I was baffled, not knowing yet about impulses.
I should written F = d mv/dt, because force is equal to the derivative of momentum, mv. Usually, mass is fixed and velocity changes, so F = m dv/dt = ma; but in the quiz problem, velocity is constant, but the mass is changing, so F = v dm/dt.
Simple, but I muffed it, and because it was simple, and because I was extremely sore at myself for muffing it, I couldn't ever forget it, so I would never make that kind of mistake again.
Curiously, this year's 8.01 quiz #2 also featured rail cars moving along a frictionless track.
I wonder if any of the freshmen will remember the problem 50 years from now. Probably just the ones who got it wrong.








Comments
Don Ward SB '6…
Wed, 12/28/2011 4:03pm
Not only do I remember that quiz, but I have thought about it from time to time over the years. It opened my eyes to what I called the "MIT mind" which looks at things in different (sometime devious) ways. I recall getting a grade on quiz #1 that was a C on the curve, but I aced #2, and snagged a 100.
Bob Kerns
Tue, 12/27/2011 2:46pm
Hah! I had no trouble deriving the formula for a rocket on the 8.01
quiz (rather than memorizing it -- something I was never good at). Basically the same problem in reverse -- shedding mass and momentum.
But it never occurred to me until just now to view the derivative of momentum as a generalization of F=ma! A missing link in my little Newtonian world-view I didn't even realize was missing!
Too bad I got it right, I guess...
Thanks!
Tom Lawford MD EE 61
Tue, 12/27/2011 2:26pm
In electronic circuit theory 6.021 years ago, the test was one question, an electronic circuit with the value of the circuit components, but with an added number, the max value of voltage or current the item could tolerate. The question was: When the switch is thrown on name the order in which the parts burn out and explain why. I mumbled when I looked at it "I had never even thought about this." Prof Gray beamed from ear to ear, and said "I know. It came to me at 2AM in the middle of the night and I was so excited I had to jump out of bed and constructed the question right then. We believe that how well you can think is more important than how well you can memorize." Of course he was later to become Chancellor. Best teacher I have ever had. When you raised your hand, he had ESP and knew exactly which of his last ten sentences had you puzzled. What a priviledge his class was
Robert V. Garvin
Tue, 12/27/2011 1:09pm
In my day, it was P=mf
The grades you could get were H, C, P, F
Besides that, my hip hurts!
Nikolaos Markis
Mon, 12/05/2011 12:58pm
Interesting article, and I have strengthened my intuition from this mistake.
Thanks,
Nick
Dave
Mon, 12/05/2011 12:35am
Is there a way to solve the problem without using calculus?